Over the past few years large cosmological simulations have been performed to determine the properties of dark matter haloes, including density profiles, shapes and accretion histories (see e.g. Bryan et al. 2013; Klypin et al. 2011; Springel et al. 2005). These properties are of particular interest, as forming galaxies depend on the structural properties of the haloes in which they are embedded.
During hierarchical growth, halos acquire a density profile with a near universal shape, that can be described by the NFW profile (Navarro et al. 1996, 1997). As discussed briefly in the previous posts, the NFW density profile is described by just two parameters, halo mass, M, and concentration, c. A halo’s concentration is defined as the ratio of the virial radius, $latex r_{200}$, and the scale radius, $latex r_{-2}$, which is defined as the radius where the logarithmic density slope is −2. Thus, given the NFW profile, only a relation between concentration and halo mass is needed to fully specify halo structure at fixed mass. Therefore, numerous studies have been undertaken to improve the concentration-mass (c-M) calibration.
Despite its importance, there is still no solid agreement on the dependence of halo concentration on halo mass and redshift. A small change in the adopted cosmology can have important effects on the structure of dark matter halos (Maccio et al. 2008), and on their mass histories (Zhao et al. 2009). For example, the mean concentrations of dwarf-scale dark matter halos change by a factor of 1.5 between the various Wilkinson Microwave Anisotropy Probe (WMAP) cosmologies (Spergel et al. 2003, 2007). The Planck cosmology (Planck Collaboration et al. 2014) has higher matter density, $latex \Omega_{0}$, and higher power spectrum normalization, $latex \sigma_{8}$, compared to the cosmological parameters of the year 5 data release of WMAP (WMAP5; Komatsu et al. 2009). The Planck cosmology therefore suggests that halos assemble earlier and are more concentrated (c.f. c − M relations from Dutton & Maccio 2014 and Duffy et al. 2008).
However, cosmology may not be the primary reason for the differences in the c-M relations found by various authors. Recent works that adopt the same cosmology still find different c-M relations (compare for example Dutton & Maccio 2014 and Diemer & Kravtsov 2015, or Klypin et al. 2011 and Prada et al. 2012). Dutton & Maccio (2014), found that the c − M relation is well described by a power-law, but flattens at high redshift and exhibits a positive slope at $latex z>4$. In contrast, Diemer & Kravtsov (2015) found a strong upturn in the high-mass end of the c − M relation at all redshifts. The disparity between these studies could be due to the dynamical state of the selected dark matter halos. For example, Ludlow et al. (2012) showed that massive halos that are substantially out of equilibrium are more likely to be found at a transient stage of high concentration, thus explaining the puzzling upturn in the high-mass end of the c − M relation. Indeed, they reported that the upturn disappears when only dynamically-relaxed systems are considered.
It is generally believed that the c−M relation relies on the fact that concentrations depend on the evolutionary stage of halos when they were formed, and thus on their mass accretion histories (MAH). In this work (Correa C. A., Wyithe J. S. B., Schaye J., Duffy A. R., 2015c, MNRAS, 452, 1217) we explore the relation between the halo MAH and its concentration. We derive a semi-analytic, physically motivated model for dark matter halo concentration as a function of halo mass and redshift. The semi-analytic model combines an analytic model for the halo MAH derived in Correa et al. (2015a), with the empirical relation between concentration and formation time obtained in Correa et al. (2015b). The model predicts a change of slope in the $latex z\sim0$ c−M relation at a mass scale of $latex 10^{11}M_{\odot}$. We find that this is due to the change in the functional form of the halo MAH, which goes from being dominated by an exponential (for high-mass halos) to a power-law (for low-mass halos). During the latter phase, the core radius remains approximately constant, and the concentration grows due to the drop of the background density. We also analyse how the c − M relation predicted by this work affects the power produced by dark matter annihilation, finding that at z = 0 the power is two orders of magnitude lower than that obtained from extrapolating best-fitting c − M relations. Finally, we provide fitting formulas for the c − M relations, a step-by-step description on how to implement the semi-analytic c − M model in the Code page.
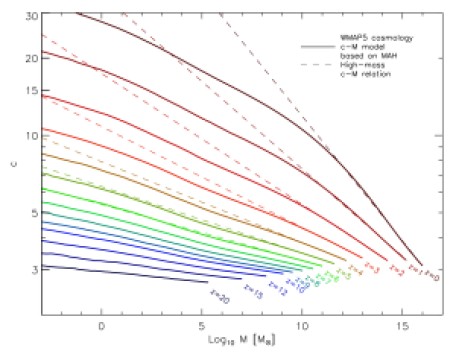
The figure shows the concentration – mass relation obtained by the semi-analytic model for the WMAP5 cosmology over a wide range of halo masses $latex (log_{10}M/M_{\odot}=[-2,16])$ and redshifts ($latex z = 0-20$). The lines are coloured as a function of redshift as indicated. The dashed lines correspond to power-law fits to the high-mass c − M relation.